Impossible problems | science game

A typical light bulb that turns on and off from two switches (one of which fails repeatedly, causing inconvenience of going back and forth from one switch to another until the light turns on), mentioned last week, operates according to the figure diagram.

When both switches are connected at A, or both are connected at B, the circuit is closed and the lamp lights up; Otherwise, the circuit is cut off and the lamp goes out. (In the diagram, everything looks very simple; it is another thing to properly connect the cables in a real installation).

Our regular commentator Luca Tanganelli takes the question a step further and asks what the circuit would look like if the lamp itself was turned on and off from three switches. What if we generalize the problem to a lamp with n switches?

For the unknown switch light, this is Vicente Pardo’s solution:

“You flip the first switch and you wait (let’s say three minutes), you turn it off and you flip the next switch, then you go up to the first floor. Three possibilities: The light bulb is on, confirming the second switch you just flipped; if the light is off, go and touch it with your hand And if it’s hot it’s the first switch I’ve turned on for three minutes, and if it’s cold it’s the third switch.”

One tip: If you wait three minutes, don’t go upstairs, or if the good key is the first key, you’ll burn your hand.

Back to engineering

After a few weeks of touring physics and its surroundings, and before the purists insisting that this is a math department get upset, let’s go back to engineering with two seemingly impossible problems, one due to lack of data and one due to overabundance.

Featured first in Martin Gardner’s Brilliant Fun Mathematics section of Scientific American:

Divide an obtuse triangle into acute triangles or show that it is impossible.

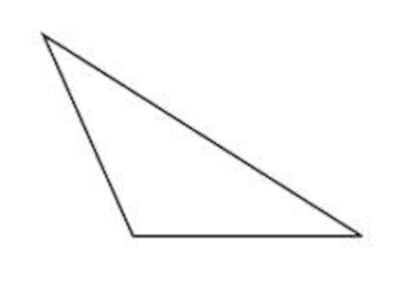
The second I saw in one of Clifford Pickover’s highly recommended books (I don’t remember any; some readers probably know) and although in its original version it had something to do with a spherical spaceship and a clawed alien, in short, it says like this:

The area and volume of a sphere are both a four-digit integer multiplied by π. What is the radius of the sphere?

Since we have a ball in hand, let’s imagine that it is filled with a gas whose molecules are moving inside. Will there be a level at all times cutting the ball such that there are the same number of particles on each side of it? (The number of particles inside the sphere is assumed to be the same, of course.)

By the way, this installment of science game Posted on 12 11 21. What can seasoned readers say about the elegant analogue 121121?

Carlo Frappetti Writer, mathematician, and member of the New York Academy of Sciences. He has published more than 50 popular scientific works for adults, children and youth, including “Damn physics”, “Damn maths” and “The great game”. He was a screenwriter for “La bola de cristal”.

You can follow Thing employment Facebook social networking siteAnd Twitter NS Instagram, or sign up here to receive Weekly newsletter.